Psychology
Can a Statistic Used in Psychology Reveal the True Home Run Champ?
Z-scores can determine whether Judge, Bonds, or Ruth is the real champ.
Posted October 8, 2022 Reviewed by Vanessa Lancaster
Key points
- This sseason, Aaron Judge broke Roger Maris' American League home run record, though Barry Bonds still holds the overall MLB HR record.
- Since Barry Bonds allegedly used steroids, but there's never been suspicion about Judge using steroids, some consider Judge the true HR champ.
- Steroids are just one factor that affects our bias of baseball performance and the sport's statistics each season.
- Z-scores, which are used in psychological testing, control for many biasing factors across seasons and thus can reveal baseball's true HR champ.

All rise: Aaron Judge is the new American League home run champion! But how does his accomplishment compare with the record-setting home run (HR) performances of the past?
Aaron Judge, the recently-named league MVP, hit 62 HRs this year, eclipsing the American League (AL) record set by Roger Maris in 1961. The Major League Baseball (MLB) record, set by Barry Bonds in 2001, remains at 73. When Bonds broke the MLB record 21 years ago, many judged his feat to be illegitimate, as he and most of his peers reportedly used steroids.
Today, however, with more rigorous drug testing in baseball, fans and insiders believe that steroid use is greatly reduced. Since there have never been suspicions about Aaron Judge using steroids, many people feel that he, not Bonds, is the "true" or "clean" HR champ.
Despite this, no one would deny, even Judge's most fervent fans, that it's much easier to hit home runs today than it ever was, as ballparks are much smaller and the baseballs are wound tighter than ever. With these and other factors making it easier to hit HRs now than in most previous eras, is there a way to fairly compare the HR totals of current players, like Aaron Judge, with those of the past, like Roger Maris and Babe Ruth?
Can a statistic used in psychology–the z-score–deliver a verdict?
In 2016 I was invited to give a presentation at the National Baseball Hall of Fame on this very topic: it was a talk based on a book, Z-Score: How a Statistic Used in Psychology Will Revolutionize Baseball, that I co-authored the previous year with my colleague, Jason Wirchin.
What the "ƒ" is a z-score?
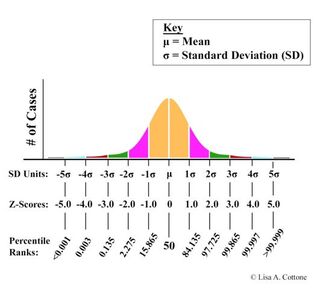
A z-score is a type of standardized statistic (another type is the percentile) that describes an individual’s performance relative to the performance of all others in the same group. When converting raw scores from any data set to z-scores, the process automatically controls for countless factors that might influence the whole group of scores. For example, whether the players in any given season were using steroids or whether the baseballs used that season were irregular in some way.
This is because inherent within every z-score is information about two important components of the data set from which it came. One component is the mean or average score of the group (e.g., the average number of home runs hit by AL players in 2022), and the other is the standard deviation (SD) of the group, which describes how far apart the scores of a data set are from the mean.

As illustrated here, z-scores are computed by subtracting the mean of a distribution from a particular score and then dividing it by the data set's standard deviation.
Z-scores are computed in such a way that the mean score of any data set will always convert to a z-score of 0, and all raw scores below the mean will convert to z-scores with negative values (e.g., -1, -2, -3, etc.), while raw scores above the mean will convert to z-scores with positive values, outward to infinity (e.g., +1, +2, +3).
For those who might be interested in knowing more about what a z-score actually is, I invite you to check out the first chapter of my book, provided for free here, or a video of my presentation at the National Baseball Hall of Fame.
Z-scores in Psychology
In psychology, z-scores are instrumental, particularly within cognitive testing and neuropsychological evaluations, as they afford us several advantages over examining raw scores of human performance. First, converting raw scores to z-scores (or another standardized statistic, like percentiles) allows us to compare all measures on the same metric.
In neuropsychological testing, individual tests yield raw scores in many metrics, like the number of items correctly answered on an IQ test; the time needed to complete a maze; and the number of pegs fitted into a grooved pegboard in a minute, among others.
But when we convert raw scores of all types into standardized scores, like z-scores and percentiles, we can more easily compare apples to apples because everything is on the same metric: the standardized score. In addition, standardized statistics also provide important context for each score, containing within it information about the average performance (i.e., the mean score) on that test.
Who's the Real Home Run Champ?
In baseball history, there are a few seasons that stand out among all others because of the singular home run total that the home run champion had during that season. The first of these seasons is 1919. Although that season is most often associated with the so-called Black Sox Scandal that marred the World Series, it is also the season in which the first significant home run total was established. It was in 1919 that Babe Ruth hit 29 home runs, not with the New York Yankees but with the Boston Red Sox.
Babe Ruth spent much of his time that season as a pitcher, but he still hit nearly three times as many home runs as the next closest player, nearly ten times the league average. His performance was so freakishly extraordinary that it led to the most infamous sports transaction of all time: the Red Sox sale of Babe Ruth's contract to the Yankees for $100,000, a record sum at the time, to reportedly help then-Red Sox owner Harry Frazee finance his upcoming Broadway show (Reisler, 2004). Babe Ruth's 29 HRs (league mean = 3.47; league SD = 4.48) in 1919 yielded him a z-score of 5.70.
A year later, in 1920, in Babe Ruth's first season with the Yankees, he nearly doubled his gargantuan home run total from the year before, hitting 54 HRs (league mean = 6.49; league SD = 8.33). However, HRs were becoming more popular around the league for several reasons, and the league average also nearly doubled, jumping from 3.47 to 6.49. Babe Ruth's 54 HRs in 1920 produced an even higher z-score than his 29 HRs the year before, but not by much: 5.71.
The 1927 Yankees are often regarded as the best team in baseball history, featuring a lineup nicknamed Murderer's Row that included Lou Gehrig and Tony Lazzeri, among others. In this historic season, Babe Ruth had the highest single-season HR total of his career, 60, but since HRs were continuing to grow around the league, this singular HR total did not yield the highest of Babe Ruth's z-scores. His z-score for this season was a mere 4.71.
Babe Ruth's 60 HRs stood as the MLB record for 34 years until 1961 when Mickey Mantle and Roger Maris raced to erase it. Mickey Mantle got hurt, missing several weeks of the season, and this allowed Roger Maris to be the one who eclipsed Ruth's record, hitting 61 HRs (league mean = 18.31; league SD = 14.23) that year. Maris, however, had a considerable advantage over Ruth as MLB expanded the schedule from 154 games to 162 games in 1961.
However, everyone in the AL that season enjoyed this advantage, not just Maris. That's why z-score comparisons are superior to raw score comparisons when examining statistics across eras in sports: they account for differences that affect entire cohorts from year to year.
Despite Roger Maris' 61 HRs being greater than any of Ruth's totals, his z-score for this record-breaking total was just 3.00, less than Ruth's top three totals.
The year 2001 was notable for many things, not the least of which was the 9/11 attacks on America. But it was also the year Barry Bonds set the current MLB HR record, hitting 73 homers (league mean 22.42; league SD 14.79), breaking the record Mark McGwire set in 1998. Bonds' record still stands but many regard it with suspicion, as numerous reports, including the investigative book Game of Shadows, linked Bonds with steroid use.
However, according to other reports, most other players in that era also used steroids, giving them the same advantage as Bonds. Once again, z-scores account for this because they are computed using each respective data set's mean and standard deviation (e.g., the cohort of MLB players in 2001). Despite posting a mythical total of 73 HRs, Barry Bonds' z-score for the 2001 season was just 3.42, which was lower than the z-score for Ruth's top three HR seasons but more than Roger Maris' z-score in 1961.
The Verdict
How does Aaron Judge's record-setting HR total this season rank with the others? This year, Judge's 62 homers (league mean = 19.52; league SD = 10.14), which broke Roger Maris' AL record of 61, yielded him a z-score–4.19. This z-score was greater than Roger Maris' z-score for HRs in 1961 and higher than Barry Bonds' z-score for his Major League record 73 HRs in 2001.

Though many players have eclipsed Babe Ruth's raw HR totals for those seasons, most recently Judge, Ruth still has the three highest z-scores for home runs (with his z-score of 5.71 for the 54 HRs he hit in 1920 being the highest of all time): hence, it is Babe Ruth, not Aaron Judge or Barry Bonds, that is the true, z-score Sultan of Swat!
References
Reisler, J. (2004). Babe Ruth: Launching the Legend. New York: McGraw-Hill. ISBN 978-0-07-143244-3.




