Trust
Trust and Power in the Ultimatum Game
The mind begins where the math ends.
Posted March 13, 2020

Ultimare (Latin), verb: to come to an end
The simplest form of bargaining involves an ultimatum and a response. One person makes a proposal, e.g., to sell a widget for the price of $X, and another person agrees and pays, or walks away. The presumption is that if a deal is struck, an exchange occurs that leaves both parties better off. A walk-away is likely inasmuch as the seller’s asking price is high and the client’s tolerance thereof is low.
The famous Ultimatum Game dramatizes this situation (Güth et al., 1982). In its experimental form, this game neutralizes the usual real-world economic constraints. One person, the designated proposer, receives free cash, typically $10, to be shared with another person, the receiver. Classic game theory predicts that the proposer offers the smallest amount possible to the receiver, and that the receiver accepts it. Professor Güth foresaw the failure of this prediction. Indeed, the modal offer is $5, the average about $4, and offers of $2 or $1 are typically rejected. When the receiver rejects an offer, neither player gets any money, which makes such a veto a costly signal to, and perhaps punishment of, a greedy proposer. Many receivers rather have nothing than be competitively exploited.
Most studies focus on the psychology of the receiver and neglect the psychology of the proposer. The receiver’s veto is of great interest; it is often understood in moral terms, even to the point of being interpreted as an act of altruism. A veto might help the proposer to be a better person next time, even if the next ultimatum involves a different receiver. This focus on the tough receiver, important as it might be, leaves out other interesting aspects of the ultimatum game.
Trust
In experimental economics, interpersonal trust is modeled with the trust game (Evans & Krueger, 2009). The first player, the trustor, receives $10 and is free to either keep it or to transfer it to the second player, the trustee, in which case the amount triples. If there is a transfer, the trustee can keep the $30 or split it with the trustor. The investment version of this game is similar to the ultimatum game in that the transferred amount provides psychological information about the trustor to the trustee. This similarity leaves an empirical footprint. The less the trustor invests, the less (in proportionate terms) the trustee returns – much like lousy offers in the ultimatum game tend to be rejected.
In the ultimatum game, but not in the trust game, the pie is fixed. How then does the ultimatum game involve trust? The proposer makes an offer thought to be good enough for the receiver to accept it. The proposer thus trusts the receiver not to veto the deal – which would be to the material detriment of both. Whereas in the trust game, the trustor’s investment creates wealth, trust in the ultimatum game seeks to create inequality. The proposer who offers the smallest amount to the receiver is, on the face of it, the most trusting. The receiver presumably trusts the proposer to offer a good deal (note that in the trust game, each trustee is also a trustor, trusting to receive a good investment). The receiver’s noncooperative choice (veto!) destroys everyone's wealth in the ultimatum game, whereas the trustee’s noncooperative choice (betrayal) in the trust game only destroys the trustor’s wealth.
We have seen that for the proposer in the ultimatum game, a strong propensity to trust contravenes the social values of fairness and benevolence – perhaps a surprising result. For the receiver, trust entails expectations of fairness or benevolence, which, if dashed, promote retribution.
Power
Power, you might think, is antithetical to trust. If you have power, you don’t need to trust; if you have no power, trust is all you have – or so intuition says. Indeed, this intuition may often be valid, but there is more to learn. In the ultimatum game, trust and power are positively related when we compare the two players. To the proposer, trust is a more relevant consideration when deciding on the size of an offer than it is for the receiver when deciding whether to reject it. The proposer has arguably more power than the receiver because the proposer has a good chance of going home with more money than the receiver (e.g., a $6 to $4 split is rarely vetoed). Within the proposer’s role, trust and power go together at the time the offer is made. A proposer who offers $1 to the receiver evidently shows more trust and feels more powerful than a proposer who offers $5.
For a closer look at the power dynamics in the ultimatum game, consider a variant in which both players record their choice simultaneously. The proposer submits an offer and the receiver commits to either accepting or rejecting it before having seen it. Now the ultimatum game is an ultimatum dilemma. The proposer might think that a receiver would not reject an offer without having seen it, and then offer very little. A receiver might anticipate this line of thought and preemptively reject the offer – a line of reasoning that the proposer in turn could anticipate – and down into the infinitely regressing metacognitive rabbit hole.
Stepping away from this rabbit hole, we can anticipate the two players’ preference rankings and explore the implications of their intersections (Brams, 2011). The proposer and the receiver have two options each, one harsh, H, and one soft, S. The proposer can harshly offer $1 to the receiver or softly offer $5. The receiver can harshly reject an offer or softly accept it.
Surely a proposer will think it best to have a low (harsh) offer accepted, HS. Let’s assign this a value of 4. Having a generous offer accepted, SS, is the next best outcome (3). It would be worse to have a generous offer rejected, SH (2), and it would be the pits, for loss of a great gain, to have a poor offer rejected, HH (1). In other words, the proposer ‘s first interest is for the receiver to have a soft strategy of acceptance. But if the receiver accepts, the proposer prefers the harsh strategy of offering less; if the receiver harshly rejects an offer, the proposer would prefer having offered more, that is, forfeiting less. The proposer’s second interest is to be reactive, that is, to respond harshly to a soft receiver, and to respond softly to a harsh one.
The receiver most prefers to accept a good offer, SS (4), followed by the unpleasant but self-righteous rejection of a bad offer, HH (3). It would be still worse to accept a bad offer, HS (2), and the worst to reject a good one, SH (1). In other words, the receiver’s primary interest is to respond to the proposer in kind, that is, to treat a harsh proposer harshly, and a soft proposer softly. The receiver’s second interest is to act softly, as there is more to be gained materially with the strategy of acceptance.
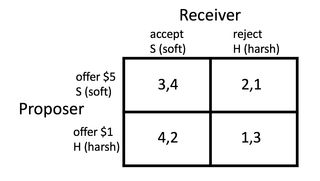
The two sets of preferences are shown in the matrix to the left. It would be collectively best if both players acted softly. The receiver would have the most one could hope for in this game, but the proposer might wistfully contemplate the loss of a larger share. This is why the soft-soft, SS, outcome is not a Nash equilibrium. The proposer, if given the chance, might want to switch to a harsh, H, strategy to gain one preference rank while taking 2 from the receiver. The HS outcome, in turn, motivates the receiver to switch from S to H, yielding the HH outcome. The receiver gains 1 point while the proposer loses 2. The twirl continues with the proposer now switching back to S, gaining 1 point and taking 2 from the receiver. But SH is the most dismal outcome overall, and at this point the receiver can make the greatest difference by switching from H to S. The receiver gains 3 points, while the proposer gains 1. It is the only switch that benefits both players and that benefits the switcher more than the other.
This last move, and the fact that the receiver ends up with his or her or their first preference, suggests that the receiver has more power than the proposer, a result that contrasts with the conclusion reached for the sequential game, where the proposer wields more power. Yet, the receiver’s power in the simultaneous game remains fragile; the proposer can always switch to a harsher stance (if switching is allowed). This fragility is a feature of all social power, except the kind that is squarely staked on coercion. The less powerful support the more powerful as long as it is in their interest not to rebel. Analyses of similar power games yield the same basic result (Krueger, 2014ab).
The Maharishi Yogi says that “to resolve problems through negotiation is a very childish approach.” How are they solved then? The Yogi asserts that they “will disappear as darkness disappears with the onset of light.” Were it only so! Back in the real world, we encounter one another and interact mindfully, giving thanks to game theory and psychology.
Brams, S. J. (2011). Game theory and the humanities. Cambridge, MA: MIT Press.
Evans, A. M., & Krueger, J. I. (2009). The psychology (and economics) of trust. Social and Personality Psychology Compass: Intrapersonal Processes, 3, 1003-1017.
Güth, W., Schmittberger, R., & Schwarze, B. (1982). An experimental analysis of ultimatum bargaining. Journal of Economic Behavior and Organization, 3, 367–88.
Krueger, J. I. (2014a). How Robinson Crusoe managed his Man Friday. Psychology Today Online. https://www.psychologytoday.com/us/blog/one-among-many/201402/how-robin…
Krueger, J. I. (2014b). A general power game. Psychology Today Online. https://www.psychologytoday.com/us/blog/one-among-many/201402/general-p…




