
I'd luv to kiss ya, but I just washed my hair. ~ Bette Davis
A dilemma presented itself at lunch. Should I order wine – e.g., a glass of delicious Ribera del Duero – or not? Ordering wine with lunch at the Faculty Club is uncommon but not unheard of. In the soft-boiled puritanical atmosphere of a private East Coast college, sitting down with a high-ranking host, the choice turns out to be tricky (for him too, as we shall see). Suppose both of us would enjoy a nice RdD. I am worried that I might be seen as presumptuous or lushy; he (being higher ranking) is mainly concerned about the latter. If we both ask for water or tea, we both feel ok, though oddly unfulfilled. If one of us orders wine while the other does not, the wine drinker feels worst because of the embarrassment, while the water drinker has at least sidestepped the faux pas.
Romance can offer a similar pickle. Both want to share a kiss, but fear that the other might not. Mutual kissing is best, not kissing is frustrating but not terrible, being the one leaning in for a kiss without getting a response is horrible, while being the aloof kissee is not quite as bad. Both scenarios contain a desire to consume and a fear of being left hanging. Hence, they capture the agony of social shyness.
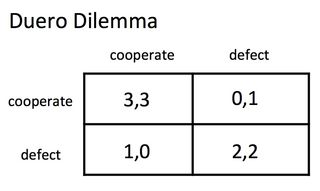
This theme can be represented as a symmetrical interpersonal dilemma or game, in which the payoffs are ranked as follows: mutual cooperation (wine; kiss) = 3 hedons (pleasure points) > mutual defection (water; gaze only) = 2 hedons > unilateral defection = 1 hedon > unilateral cooperation: 0 hedon. The payoff matrix shows these arbitrary but instructive numbers.
The Duero Dilemma is a type of coordination game. If you knew what the other person will do, you’d do the same. But you don’t, hence the dilemma. Some might say that mutual cooperation (wine, kissing) is a clear attraction; it is a Schelling point drawing attention. Yet, many of us will hesitate to cooperate (order wine; come in for a kiss) because we are not convinced the other person will do the same, and if they don’t, unilateral cooperation is the worst thing that can happen. The appeal of defection (water and kisslessness) is that it avoids the worst possible outcome. It preserves a minimum of dignity. Much as we might say we ourselves are not so concerned with this sort of loss aversion, we can’t be sure that the other person is not, and therefore we lose our moxie.
What does game theory say? Besides the two pure Nash equilibria, mutual cooperation and mutual defection, there is a mixed-strategy equilbrium. If I cooperate with a probability of .5, you will be indifferent between cooperation and defection. Likewise, if you cooperate with probability .5, I am indifferent, and so – so game theory – we both flip a mental coin to ‘decide.’ Though considered the rational solution by game theorists, this coin flipping does not appear to yield much good. It literally leaves things to chance, and each possible outcome, from the most hoped for to the most dreaded, is equally likely.
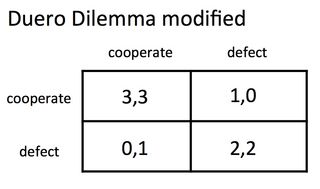
Now consider a modified Duero dilemma (payoffs in the second matrix). The only change is that unilateral defection is now worse than unilateral cooperation. The water drinker and the kiss shirker feel lousier than the wine drinker or the would be kisser. The wine drinker has at least the wine to enjoy, and the would be kisser has the solace of having tried. Notice that the sum of the two cooperation payoffs is now greater (4) than the sum of the two defection payoffs (2). Yet, the best strategy is still to do whatever the other person is doing, if you only knew what that was. Not knowing, game theory asks you to cooperate with the Nash equilibrium strategy of p(cooperation) = .25. In this modified game, cooperation is a bit more likely because drinking wine alone or having a kiss unreciprocated are not the worst things that can happen. With this preference structure, you can live a little more boldly.
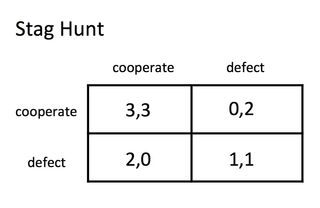
Both, the original Duero dilemma and its modification, are mutations of the canonical assurance game, or stag hunt (see payoff matrix). In the stag hunt, wining or kissing alone are the worst, as they are in the original Duero. Yet, unilateral defection (water, kisslessness) is second best, which means the stag hunt represents a strong model of shyness. Again, though, notice that the summed payoff for cooperation is the same as the summed payoff for defection, which means the rational game theorist flips a coin.

Finally, suppose you didn’t know the other person’s preferences. Knowing only your own (3 for mutual cooperation, 2 for mutual defection, 1 for unilateral defection, and 0 for unilateral cooperation) you will probably be indifferent out of ignorance and flip a coin, or, if you want to err on the side of caution, defect. Would that be so whatever the other’s payoffs are? No. Consider an asymmetrical Duero, where only your payoffs (you are the row player, so your payoff is to the left of the comma in each cell) are those of Duero. Your partner’s payoffs are those of the prisoner’s dilemma, i.e., unilaterial defection > mutual cooperation > mutual defection > unilateral cooperation. If you knew that person’s preferences, you would defect, at least reaping the satisfation of finding your second best payoff, whereas the other has to settle for the second worst. If you flip a coin, your two worst outcomes will come to pass with the same probability.
What can we (I) advise you to do? The lofty voice says: Live boldly, have that glass of wine and kiss the guy (girl, trans, person). Experience says: I did not have that wine at the faculty club. I had some sort of orangish herbal brew. Loss aversion is a terrible thing.


